Simon Singh, Yuji Okitani and Matt Parker
Last November, we hosted the final of the UK’s Who Wants to Be a Mathematician competition, which was won by Yuji Okitani from Tapton School in Sheffield. Yuji will be competing in the grand final this weekend, against the best young mathematicians in the US and Canada.
While we wish Yuji the best of luck in the competition, we thought we’d give you the opportunity to test yourself against the questions Yuji faced in the final. How would you have fared?
Question 1.
Which of the following numbers is divisible by 3?
| A) 33331 | B) 33311 | C) 33111 | D) 31111 | E) 11111 |
Check the answer:
1-C) 33111
Question 2.
A set of boxes of different heights must be packed into bins, each of which is 10 units tall. The boxes are 1 unit wide and 1 unit deep, fit into the bins exactly, and must be stacked vertically inside the bins. If the boxes are of heights 2, 2, 4, 4, 5, 6 and 7 units, what’s the minimum number of bins needed to fit all the boxes?
| A) 2 | B) 3 | C) 4 | D) 5 | E) 6 |
Check the answer:
2-C) 4
Question 3.
How many faces does a convex polyhedron with 120 edges and 60 vertices have?
| A) 60 | B) 62 | C) 80 | D) 84 | E) 92 |
Check the answer:
3-B) 62
Question 4.
This grid must be filled so that the numbers 1-5 each occur exactly once in each row, column and the two main diagonals.
What is the sum of the squares in grey?
| A) 13 | B) 11 | C) 12 | D) 15 | E) 9 |
Check the answer:
4-B) 11
Question 5.
Who was the first woman to win a Fields Medal, the most prestigious prize in mathematics – “the Nobel Prize of maths”?
| A) Katherine Johnson | B) Grace Hopper | C) Alice Etheridge | D) Lisa Simpson | E) Maryam Mirzakhani |
Check the answer:
5-E) Maryam Mirzakhani
Question 6.
A fair die has the numbers -3, -2, -1, 0, 1, 2 , and 3 on its faces. If the die is rolled twice, what is the probability that the sum of the two numbers is zero?
| A) 6/49 | B) 1/7 | C) 1/2 | D) 1/14 | E) 8/49 |
Check the answer:
6-B) 1/7
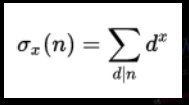Question 7.
The sigma function, given here, is the sum of the divisors of a number each raised to the power x. What is σ2(12)?
| A) 174 | B) 200 | C) 209 | D) 210 | E) 212 |
Check the answer:
7-D) 210
Question 8.
A goat is tethered by a 25m rope to the outside corner of a 20m by 15m shed. What is its total grazing area (in square metres)?
| A) 400π m² | B) 425π m² | C) 500π m² | D) 505π m² | E) 525π m² |
Check the answer:
8-C) 500π m²
Question 9.
A factorion is a natural number that equals the sum of the factorials of its digits. One of these numbers is a factorion. Which one?
| A) 624 | B) 32 | C) 145 | D) 205 | E) 154 |
Check the answer:
9-C) 145
Question 10.
Suppose a cube has side lengths of 1.5 cm. From one corner of the cube a shortest path is drawn on the surface of the cube to each of the other seven corners. What is the total length (in cm) of the seven paths?
| A) 9+3/√2 | B) 12 | C) 6+3/√2+√5/2 | D) 4.5+3√2.25 | E) 4.5+9/√2+3√5/2 |
Check the answer:
10-E) 4.5 + 9/√2 + 3√5/2
Question 11.
What is the name given to this sequence of numbers? The first three terms have been omitted. 20, 35, 56, 84, 120, 165, 220
| A) Fibonacci numbers | B) Happy numbers | C) Tetrahedral numbers | D) Sophie Germain primes | E) Mersenne numbers |
Check the answer:
11-C) Tetrahedral numbers
Question 12.
Which of this problems has the answer YES?
| A) Can you cross all the Bridges of Konigsberg? | B) Can you move the discs in the Towers of Hanoi? | C) Can you solve the Utilities Problem? | D) Can you place 31 dominoes on a Mutilated Chessboard? | E) Can you visit all rooms in the Five Rooms Puzzle? |
Check the answer:
12-B) The Towers of Hanoi
Question 13.
This year the Bakhshali manuscript appeared to show a record of zero approximately 500 years earlier than previous historical estimates. The Bakhshali manuscript originated from:
| A) Middle East | B) Europe | C) Central America | D) Indian Subcontinent | E) Far East |
Check the answer:
13-D) Indian Subcontinent
Question 14.
How many four-digit integers (between 1000 and 9999) have digits whose sum is 6?
| A) 20 | B) 36 | C) 44 | D) 56 | E) 84 |
Check the answer:
14-D) 56
Question 15.
In base n, n > 1, (100n)² represents:
| A) n² | B) n³ | C) n⁴ | D) n⁵ | E) n⁶ |
Check the answer:
15-C) n⁴
Question 16.
What proportion of three-digit numbers have at least one zero in them?
| A) 19/900 | B) 19/100 | C) 9/100 | D) 1/10 | E) 271/1000 |
Check the answer:
16-B) 19/100
Question 17.
Which of the following numbers is largest?
| A) 10! | B) 22⁴ | C) 44² | D) π million | E) 2017² |
Check the answer:
17-E) 2017²
Question 18.
When the expression (x+1)(x+2)(x+3)(x+4)(x+5) is multiplied out and like terms are collected, how many of the powers have coefficients that are divisible by 5?
| A) 1 | B) 2 | C) 3 | D) 4 | E) 5 |
Check the answer:
18-D) 4
Question 19.
Two circles of the same radius r overlap such that the edge of each passes through the other’s centre. What is the area of the region common to both?
A) |
B)  |
C)  |
D)  |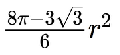
E)  |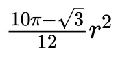
Check the answer:
19-C)
Question 20.
An Euler Brick is a cuboid where the lengths of all three edges and the diagonal of each face is an integer. If two of the edges of an Euler Brick measure 160 and 231, what is the length of the third edge?
| A) 536 | B) 601 | C) 792 | D) 801 | E) 1014 |
Check the answer:
20-C) 792
